Thomas Skidmore to Thomas Jefferson, 18 August 1822
From Thomas Skidmore
Newyork 18th Augt 1822.
Sir,
To know how to achieve the grandest object that the mind of man can contemplate, and not to have the means of achievement; to speculate on the sublimest spectacle as a mathematical certainty, &1 to want the aid that can make it a physical one, appreciable by the grosser senses of our race, as well as by persons of refined intellect; such a situation is, surely, not to be envied, if, indeed, it is not to be commiserated. Feelings which I will not attempt to describe, but which originate from a mind placed in such circumstances, and which I believe you will justly estimate, prompt me to lay before you the elements of a discovery, which I think I have made, in the construction of telescopes, and to which I conceive, the whole history of man, in point of importance, or of magnificence of result, is unable to furnish us with a parallel.
I know that your advanced age, and the retirement from the world which the infirmities it usually brings along with it, have made sacred and inviolable, ought to admonish me to abstain from this intrusion;—but how should I pardon myself, for saving to you the trouble of the perusal of this letter, and the reflections to which it may give origin, if, as the price of this forbearance, so great a delay should (possibly) arise, in the accomplishment of my (perhaps well founded) views, as to postpone to a period beyond the termination of your life, the completion of an instrument so dear to a philosopher as that must be, which could exhibit to his astonished eye, the physical properties of the moon, and even of the most distant planets and their satellites, in as distinct, and clear, and true a light, as if they were actually brought within a few yards or even a few feet of our naked eyes?
And I trust, that this invasion, which I now take the liberty of making upon your retirement, will find, if not the forgiveness, at least, the palliation, of your judgment, in what you will discover to be the fact, that the principles on which the truth of the supposed discovery rests, are very few and extremely simple, and also in the familiar acquaintance you probably have, with the principles themselves.
If the conception of an optical machine which is supposed to be capable of producing such extraordinary effects, originate in the mind of one who has no resources with which to verify, physically, the truth of such conception; there seems, for him, but one alternative, and that consists in exhibiting it, intellectually, to those whose mental qualities are such as to enable them, to predict, from its conformity or non-conformity with truths already demonstrated, how far it may be proper to afford to it, approbation or patronage. The realization, then, in my own instance, of this alternative, forms a prominent motive of the freedom I have taken in forwarding to you this communication; &, if the circumstances, before mentioned, shall not have made it improper in me, I cannot but hope, that, directly, or indirectly, the effort I am making in behalf of my proposed instrument, will not have been made, if worthy of it, without some success.
Let me proceed, then, to explain the principles of the proposed instrument. It is a telescope entirely of the reflecting kind. It is therefore, totally exempt from the evils, always more or less formidable, attendant upon refraction. It may then be expected that if the image be made upon the retina of the eye, from a surface of the proper curvature and truth of figure, we shall see our object as it actually is. This, you will agree with me, is a great desideratum.
But, I should preface my description, with a simple statement of what all optical writers, from the predecessors of Sir Isaac Newton, down to the present time, agree upon, as facts established by experience.
The eye, say they, is so constituted, that at a certain distance, say from 6 to 10 or 12 inches from it, (different in different persons), an object, for instance a page of printed letters, appears to give the greatest ease and perspicuity of vision. At a greater distance the object becomes indistinct; at a nearer distance, the letters appear to be doubled or blurr’d, as the common expression is. No other than the most advantageous is considered as the natural distance; and this latter distance is made, when they speak of the powers of magnifying instruments, the standard of comparison.
Thus, in the adjacent figure, 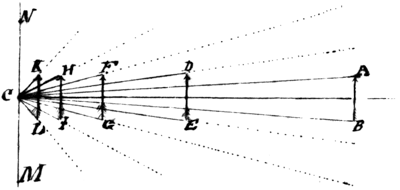 let C and arrow AB, be supposed to be equal to the natural distance of some certain person, say 10 inches. Now, were the constitution of the eye, such as to allow the arrow at AB, to be brought to DE, say 5 inches from the place of the eye, C; & still to afford distinct and clear vision, this arrow, would appear to be of double the length which it has at AB. The angle ACB, is said to be the angle of vision under which we view the arrow, at 10 inches from the eye. The angle DCE, would also be the angle of vision of the same arrow, when placed between DE, if distinct vision could be had of it there. Remove the arrow now to, between FG. 2½ inches from the eye C, &, as before, if distinct vision could be had of it there, it would have a length compared to the same arrow at AB, as 10 inches are to 2½ inches, that is as 4 to One; since it is 4 times nearer to C, than is AB. Its angle of vision also, would correspond with the apparent increase of its length, as is shewn by the letters FCG. Again, place the arrow between HI, half as far from C, as FG is, that is, 1¼ inches. The apparent length of the arrow in this position, will be to the same arrow, when at AB, the natural distance, as 10 inches are to 1¼ inches; that is, as 8 to 1: and the angle of vision, if it could be distinct under such circumstances, would be shown by the letters HCI, corresponding to the enlargement of the arrow in its present position. Last of all, remove the arrow to between KL, taking again half the last distance from the eye C, that is, at ⅝ths of an inch from it, and the arrow, in its new position, if it could be clearly seen there, would appear to be magnified above its natural size, in the ratio of 10 inches to ⅝ of an inch; that is, as 16 to 1; so that, if the arrow were actually an inch in length, in its natural view, under the present, or a similarly magnified aspect, it would appear to be 16 inches long. And, if we consider the arrow as having breadth or thickness also, the same ratio of enlargement would take place.
let C and arrow AB, be supposed to be equal to the natural distance of some certain person, say 10 inches. Now, were the constitution of the eye, such as to allow the arrow at AB, to be brought to DE, say 5 inches from the place of the eye, C; & still to afford distinct and clear vision, this arrow, would appear to be of double the length which it has at AB. The angle ACB, is said to be the angle of vision under which we view the arrow, at 10 inches from the eye. The angle DCE, would also be the angle of vision of the same arrow, when placed between DE, if distinct vision could be had of it there. Remove the arrow now to, between FG. 2½ inches from the eye C, &, as before, if distinct vision could be had of it there, it would have a length compared to the same arrow at AB, as 10 inches are to 2½ inches, that is as 4 to One; since it is 4 times nearer to C, than is AB. Its angle of vision also, would correspond with the apparent increase of its length, as is shewn by the letters FCG. Again, place the arrow between HI, half as far from C, as FG is, that is, 1¼ inches. The apparent length of the arrow in this position, will be to the same arrow, when at AB, the natural distance, as 10 inches are to 1¼ inches; that is, as 8 to 1: and the angle of vision, if it could be distinct under such circumstances, would be shown by the letters HCI, corresponding to the enlargement of the arrow in its present position. Last of all, remove the arrow to between KL, taking again half the last distance from the eye C, that is, at ⅝ths of an inch from it, and the arrow, in its new position, if it could be clearly seen there, would appear to be magnified above its natural size, in the ratio of 10 inches to ⅝ of an inch; that is, as 16 to 1; so that, if the arrow were actually an inch in length, in its natural view, under the present, or a similarly magnified aspect, it would appear to be 16 inches long. And, if we consider the arrow as having breadth or thickness also, the same ratio of enlargement would take place.
One thing, in this little sketch of elementary principles is quite obvious; and it is to this obvious fact, that I beg your particular attention. It is this, that when the arrow is removed from AB to DE, or to FG, or to HI, or to LK, an enlargement of the angle of vision, also takes place, concurrently with & inseparably from an increase in the length of the arrow; and that one event cannot take place without the other; and that whereever an augmentation of the angle occurs, we may without mistake, or fear of mistake, infer that a corresponding increase of the length and breadth of an object which stretches across the said angle of vision, will also take place. So, again, whenever an apparent enlargement of the dimensions of an object takes place, it is equally fair, to infer that this enlargement is produced by viewing it, under an enlarged angle of vision.
It will be obvious, therefore, other circumstances being equal, that this will be the greatest magnifier, which causes the rays of light flowing from an object, to converge the fastest, or what is the same thing, with the greatest angle. Thus, an object seen distinctly, under the angle DCE; will magnify more than when the same object is seen under the angle ACB2—and will be doubly magnified, since it is only half as far from the eye C. An object seen under the angle FCG, will also be magnified more than when seen under the angle DCE and in the inverse ratio of their distances from the eye C. So will it be of the same object when seen (distinctly) under the angle HCI, which being greater than FCG, produces a corresponding augmentation of the object. The angle KCL, being an angle still greater than any of the preceding, affords an increase of magnifying power corresponding with the law already named. Lastly the greatest angle under which it is possible to view any object is that of two right angles, that is, a right line, and in this case, that right line is, NCM.
It is plain, to a mathematical mind, that, the arrow in all its various positions, is the chord of the angle which it subtends; or in other words, the chord of the angle of vision. And the same mind would probably prefer, to the rule already laid down for ascertaining the ratio of apparent magnitude, a rule something like this. As the Co-sine of half the greater of the two angles of vision, is to the Co-sine of half the smaller angle of vision,* so is the natural distance (say 10 inches) to the ratio of magnitude, or in other words, to the magnifying power.
All this is predicated upon the supposition that the naked eye is possessed of properties, different from what is known to be the fact. Now, optical instruments, it is well known, all possess their valuable properties, in the one fact, that they cause the rays of light to converge very fast, that is, under great angles; whereby they are enabled, readily to enter the pupil of the eye, under such circumstances as to produce the most distinct vision.
But every optical instrument, now known, from the microscope with its spherule eye-glass, in some instances, of 1⁄200th part of an inch in diameter, to the telescope of Herschell, have, in their very structure, and ever must have, as any one may convince himself, by reading optical works, only moderately large angles of vision. Their magnifying power is therefore very much circumscribed; and for this very obvious, and (in the present state of the science of vision,) invincible reason, to wit, that they can never cause the rays of light, which, originally flow from luminous bodies in right parallel lines, ultimately to converge geometrically to the centre of a sphere, there forming a focus, and at the same time, permit the eye to approach and occupy that focus. Such an instrument has never been made; such an one I think I know how to construct. Let me proceed.
A concave spherical reflector does not converge the rays that fall into it, parallel to its axis, to the centre of the sphere, nor, indeed, to any other point or geometrical focus. They are more or less dispersed, as you are no doubt aware. It is the concave parabolical reflector, that possesses this property, and it is no other. But as there are a great variety of parabolas, as many indeed as there are cones to cut them from, their foci are in different relative positions. Some have it (the focus) within the concavity of the parabola, and, in the line of its axis, more or less distant from the vertex of the axis. One there & one only3 is which has its focus4 neither within nor without the cavity5 of the parabola, but in the plane, which may be supposed to cover its mouth, and therefore between the two.

|
N.B. This figure appears like the section of an oblique angled cone, which is not the fact. The cone is right angled. I was in too much haste. T. S. |
Another parabola, cut from a cone of a smaller relative height than the preceding, and also exhibited at our right, may have its rays converge to a point, ☉,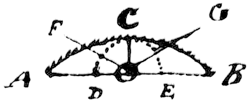 in the axis ☉C, where this axis meets the line AB, which last is at right angles with the former; and to this point all the rays of light that enter the concavity, when the axis is in a line coincident with the line of sight to, the luminous object, will be converged.
in the axis ☉C, where this axis meets the line AB, which last is at right angles with the former; and to this point all the rays of light that enter the concavity, when the axis is in a line coincident with the line of sight to, the luminous object, will be converged.
This, then, I conceive is the long sought optical machine. It is this machine which can converge, to a point the rays8 in the open air, (a medium more fit, than any we know, for the per-transmission of light), where we can have full and free access to it, as at ☉; and where their convergency to that point, is, evidently9 the same as if they proceeded at right angles from all parts of the represented semi-sphere DCE, whose radius of curvature is ☉C.
It is therefore to the point ☉, that the eye is to be applied when the greatest magnifying power which is possible, is to be sought; and the effect of such an application, if I have taken true facts, and reasoned justly from them, can amount to neither more nor less than this, that it annihilates all distance between our eye and the object we behold! This will appear, not to be doubted, when it is considered, that the co-sine of half the angle of vision (to wit; the angle or rather right line A☉B in the above figure—or 180°) is equal to nothing, or in other words has vanished. The ratio therefore of a vanished quantity, or nothing, to 10 inches the distance of natural distinct vision, is infinite—or what amounts to the same thing, the apparent remoteness of the observed object is annihilated.
Let the eye be now placed within the concavity of the reflector, and occupy a point in the axis, that point for instance where the two lines F and G meet it near the point ☉. The angle of vision in such case, would be contained between the lines F near ☉, and G near to ☉, so to speak, which is an angle much less than the angle A☉B. So that in this new position of the eye, the rays that are converged towards ☉, between A and F and between G and B are lost, since they do not enter it; and as a legitimate consequence, the magnifying power would be considerably diminished. This diminution it will be apparent, will be more or less, as the eye is carried more or less, within the concavity of the reflector.
It need scarcely be said that when we use this telescope, the back of the observer, as in Herschell’s, is towards the object viewed. It should therefore be made so large that the light intercepted by the observer’s head and the upper portion of his body, should be inconsiderable, when compared to the area, of the mouth, so to speak, of the reflector; the diameter of that of Herschell’s being four feet. This, from his experience, has been found sufficiently large, to allow a quantity of light to strike the eye, sufficient for the purposes of distinct vision. I infer, that under precisely similar circumstances, the same would take place in mine.
Now, as to the execution. To obtain the perfect parabolic form or curve, has been thought to be extremely difficult, and perhaps impossible. Mr Herschell is stated to have discovered a very useful method of obtaining the curve of the parabola, which he used; And that he keeps it a secret. For my own part, I would ask, what can be more easy, after ascertaining the kind and magnitude of the cone wanted, than to construct it very carefully conformable thereto, and as carefully also, to cut away, parallel to one of its sides in the proper point, all save the smaller portion, which will be possessed of a perfectly plane surface bounded by the parabolic curve wanted—copies of which can easily be obtained, by placing the said plane surface upon another plane surface and with a pointed instrument marking around the margin of the preserved conical fragment?
I confess that I think that where a thread or silk string, is used to obtain this curve, (as is the fact sometimes), that success is not to be expected for obvious reasons—but in such a mode as this, I discover no source of greater error or uncertainty, than there would be in attempting to draw a true circle, with a good pair of compasses. There is more labor in the process, to be sure, and that is all.
It seems evident to me, then, that the means are at hand, of obtaining the true figure. The converging of the rays, geometrically true to their common focus, may confidently be expected, as it is certainly very much to be desired where we want a perfect image. At least, I submit it to your judgment, if I am wrong.
As to the material. It may be of glass; but then it would require, before silvering, to have, both concave and convex surfaces ground parallel to each other, and this, tho’ by no means an impracticable achievement, would yet be attended with some danger of being broken. It would be exposed to the same contingency afterward. In its favor it may be urged, that it reflects extremely well; and that it is not subject to be tarnished.
We may use the speculum composition of Sir Isaac Newton. But this must then be made very heavy, for purposes of strength; it is also liable to tarnish. The weight of Herschell’s is stated at 2118 ℔s. This is an evil which should [be]10 remedied if possible.
Cast-steel, too, I think might be used. I would cause enough to be melted, under proper chemical circumstances, to make a reflector, of 4 feet diameter and ½ an inch in thickness, with a short hollow stem on the back of it, to receive an iron or other strong rod, to be used to elevate it at any angle of the horizon, and point it to the object. The weight of a cast steel one, would then be about 300 ℔s—a seventh part of that composed of what is called the speculum-metal.
Silver and platina, every thing considered, might perhaps be11 entitled to preference, over all I have mentioned, but the high price of the first, and the great difficulty there would be in procuring and fabricating the other, probably will place them out of consideration.
But the cast-steel one, and the speculum-metal one, are liable to tarnish. Let us take a clear transparent circular glass-plate, large enough to cover the mouth of the reflector and let us cement its external edge, air-tight, to it. Let there be, however, in the centre of this glass plate, a circular hole large enough to admit so much of the head as to enable the eye of the observer to be placed even a little within the focus—and let this hole be closed again, by cementing into it a hollow glass semi-globe, thro’ which the rays shall pass freely, and without refraction, since they fall on its surface at right angles. The concavity of the reflector is now filled with common air, which contains oxygen gas, a prolific source of injury to the surface of metallic mirrors. Let it be further supposed that two small holes are made into some (immaterial it is, what) part of the reflector, by means of which and other contrivances, with the injection of dry hydrogen or nitrogen or other suitable gas, the common air is driven out of the reflector’s cavity, the substituted gas, occupying its place. Any effects that might be apprehended from a variation of the pressure of the atmosphere, may be obviated by causing the contained gas, to have communication, thro’ one of the aforesaid orifices, the other being closed, with the cavity of a bottle of elastic gum or caoutchouc; which, when the internal exceeds the external pressure, will, if it be thin, as it should be, expand; and when the case is reversed, will collapse.
It is understood, probably, that the telescope I have projected requires no inclosing tube, as in the case of Herschell is indispensible. This is no trifling advantage. The weight of his, made of rolled iron, could not, from the data I calculate from, be less than about 7,000 ℔s.
There is one instance, however, in which it might, possibly, be desirable to use a tube with my telescope. When it is said, that an optical instrument magnifies to infinity, it is saying all that can be said. But if the whole moon, for instance, be magnified to infinity—it is equally certain that a part of her surface may also be so magnified—and who will say that a portion or part being made larger to such an extent, is not more magnified, than when the whole only experiences such an enlargement?
When a portion only of a luminous object is to be magnified, the remainder of it is to be excluded by some opake material and this material may be, for the tube, chiefly12 canvass, and for the covering of the end of the tube, metal, in the form of a circular plate, with a small hole cut into its centre into which might be inserted a double13 concave glass, in order to give such divergency to the incident rays as to cause them, (in manner the same as when the entire luminous object is viewed) to strike upon and spread over the whole surface of the mirror, which, by the by, under such circumstances, would require a trifling alteration of its curvatures. But so perfect, I apprehend would be the telescope in its most simple form, that I imagine that this modification would seldom be resorted to.
It would become necessary, in directing this telescope to the sun, to guard the eye against its power as a burning mirror. This protection would be afforded by colored glasses of various shades, large enough to cover the reflector’s mouth—as is now practised on many occasions of a similar kind.
It is probable that I have been tedious, and I know I have been unscientific in my mode of exhibiting the principles of optics in connection with my telescope. Nor have I explained them at all, under any apprehension that they were not much better understood by yourself than by me: but it was necessary to take some standard and acknowledged facts, and by applying them legitimately, and in a manner as extensively as the limits of a letter would allow, to ascertain if I may not have discovered a valuable combination (if combination that can be called which has, intrinsically, but one piece or part) of the elements of optical science.
The subject of the absolute cost, for which such a telescope can be constructed, I confess myself inadequate to do justice to, be the mode pursued, what it might. But from the fact, that it consists, as it were of a single piece—that it will be very light—that consequently little and simple mechanism would be wanting to enable the user to give it any direction that might be desired, I am of opinion, that tho’ the gigantic one of Herschell’s required and obtained the patronage of George the Third, and cost a great deal of money, yet that this could, without inconvenience, be embraced by the finances of many private gentlemen in this Country, to say nothing of the State or General Governments—and that after a first and successful effort should have been made, all the most respectable seminaries of science in this or any other country could command the means to purchase one. I desire You to judge how happy for our national science will that day be, when such an event is consummated.
One remark more I may make, and, I trust, with propriety; and it is this, that it may seem to be more proper to have consulted the science of this City, before consulting strangers who are remote. I propose to avail myself of both resources; but, since the yellow fever, in some measure, prevails here at present, most of our professors and other enlightened men are absent—and therefore inaccessible to me at present. To other men of science, in the various states, as I happen to know them by reputation or otherwise, I shall take the same liberty that I have taken with you, that of making known my views—and I beg that you will also make them known to any one you think proper; and I beg this, under the expectation, that ultimately, some individual or government, if my views shall be deemed to be correct, will not hesitate to bestow on the world on himself or itself, and on myself a very great benefaction; a benefaction, which, it cannot be possible to fail of justly appreciating, when it is considered that if the optical machine is actually found to possess the great magnifying powers which I attribute to it, the problem, long contested among astronomers, of the moon and other heavenly bodies, being inhabited by animated beings, must be conclusively decided.—
| Thos Skidmore |
| 43 Hester st |
| N. York |
RC (DLC); at foot of text: “Th: Jefferson Esq.”; endorsed by TJ as received 25 Aug. 1822 and so recorded in SJL.
Thomas Skidmore (1790–1832), inventor and reformer, was a native of Newtown, Connecticut. He became a teacher, working successively in Princeton and Bordentown, New Jersey; Richmond, Virginia; and Edenton and New Bern, North Carolina. Skidmore then spent a few years engaged in chemical and mechanical research in Wilmington, Delaware, and Philadelphia. He settled in New York City in 1819 and became a leading figure in its labor movement. Skidmore devised a “Hydraulic & Steam Engine,” and he published articles on Robert Hare’s compound blowpipe. An abolitionist, advocate of women’s rights, and proponent of social revolution through democratic means, in 1829 Skidmore helped establish the Working Men’s Party and published The Rights of Man to Property! Being a proposition to make it equal among the adults of the Present Generation: and to provide for its equal transmission to every individual of each succeeding generation, on arriving at the age of maturity. He soon left the Working Men’s Party to form the Agrarian Party. When Skidmore unsuccessfully ran for the state legislature in 1829 on the latter party’s ticket, he was described as a “machinist.” He died in New York City from “inflammation of the liver” caused by cholera (Amos Gilbert, A Sketch of the Life of Thomas Skidmore [1984; repr. of 1834 articles, ed. Mark A. Lause]; Edward Pesson, “Thomas Skidmore, Agrarian Reformer in the Early American Labor Movement,” New York History 35 [1954]: 280–96; Sean Wilentz, Chants Democratic: New York City and the Rise of the American Working Class, 1788–1850 [1984; repr. 2004]; New York National Advocate, 21 June 1819; DNA: RG 29, CS, N.Y., New York City, 1820; American Journal of Science, and Arts 5 [1822]: 153–4, 347–51; New York Commercial Advertiser, 28 Oct. 1829; Boston Investigator, 17 Aug. 1832).
The telescope of hershell refers to the forty-foot reflector built in 1789 by the British astronomer Sir William Herschel (). A parabolic or parabolical reflector is “made in the form of a paraboloid of circular cross-section so as to reflect parallel rays to a focus, or produce a parallel beam from a source at the focus” (). speculum composition or speculum metal is “an alloy of copper and tin used for making specula” ().
1. Manuscript: “& and.”
2. Manuscript: “ACD.”
3. Preceding three words interlined.
4. Word interlined in place of “axis.”
5. Reworked from “axis.”
6. Manuscript: “aixis.”
7. Reworked from “greater.”
8. Preceding five words interlined.
9. Manuscript: “is, evidently is.”
10. Omitted word editorially supplied.
11. Skidmore here canceled “considered.”
12. Word interlined.
13. Word interlined.
Index Entries
- George III, king of Great Britain; mentioned search
- Herschel, William; British astronomer search
- microscopes search
- mirrors; as scientific equipment search
- Newton, Sir Isaac; mentioned search
- New York (city); yellow fever in search
- optics; and telescopes search
- scientific instruments; microscopes search
- scientific instruments; mirrors search
- scientific instruments; telescopes search
- Skidmore, Thomas; identified search
- Skidmore, Thomas; letter from search
- Skidmore, Thomas; proposed telescope of search
- telescopes; T. Skidmore’s proposed search
- telescopes; W. Herschel’s search
- yellow fever; in New York City search

![University of Virginia Press [link will open in a new window] University of Virginia Press](/lib/media/rotunda-white-on-blue.png)
