Thomas Jefferson: Trigonometry Exercise, 4 December 1815
Trigonometry Exercise
| 1815. Dec. 4. | The 2. cases of all the ∠s or all the sides of a spheric. △ being given. tried by the formula supplementy to Nepier’s | ||||||||
| ° ′ |  |
suppose ag. & aeg the greater segments | |||||||
 |
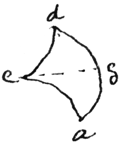a = | 49–54 | T3⁄2 × Td⁄2 of segm. of mid. p. = T5⁄2 × Td⁄2 of oppos. parts. | ||||||
| d = | 37–19 | t 31°–12′–30″ × taeg − deg⁄2 = t 43°–36′–30″ × t 6°–17′–30″ | |||||||
| e = | 62–25 | ||||||||
| ? ae = | 14–45–42 | ° ′ ″ | |||||||
| de | t. 43–36–30 | 9.9788944 | 31–12–30 | ||||||
| ag | + | t 6–17–30 | 9.0423948 | ± | 9–50–43 | ||||
| 19.0212892 | 41– 3–13 | = aeg | |||||||
| see No 91. | dg | − | t 31–12–30 | 9.7822155 | 21–21–47 | = deg. | |||
| = | t aeg − deg⁄2 = | 9.2390737 | = | 9°–50′–43″ | |||||
| for ae. | Rad × | Cos. ae = | Cot. a × Cot aeg |
| Rad |
| ° ′ ″ | |||||
| Cot. a = | 49–54– 0 | 9.9253524 | |||
| + | Cot. aeg = | 41– 3–13 | 10.0600716 | ||
| − | R = Cos. ae | < |
= 14°–45′–42″ | ||
| for de. | Rad × | Cos de = | Cot. d × Cot deg |
| Rad |
| ° ′ ″ | |||||
| Cot. d | 37–19– 0 | 10.1178993 | |||
| + | Cot. deg | 21–21–47 | 10.4076544 | ||
| − | R. = Cos de | 10.5255537. | impracticable. | ||
suppose dg & deg the greater segments
| ° ′ ″ | ||
| then (as above) tdeg − aeg⁄2 = | 9°–50′–43″ and | 41– 3–13 = deg |
| 21–21–47 = aeg |
| for ae. | Rad × | Cos. ae = | Cot. a × Cot. aeg |
| Rad |
| ° ′ ″ | |||||
| Cot a | 49–54– 0 | 9.9253524 | |||
| + | Cot aeg | 21–21–47 | 10.4076544 | ||
| − | R. = Cos. ae | 10.3330068 | impracticable. | ||
| No 89. | ||||||
 |
given | found | ° ′ ″ | |||
| d = 63–45 | dfg = | 56–26–37 | df = | 70–53–30 | ||
| e = 71–49 | efg = | 38–25–23 | ef = | 65–32–21. | or 63°–7′–30″ | |
| f = 94–52 | de = | 82–17 | ||||
| dg = | 51–56–34 | 51–56–34 | ||||
| eg = | 34–27 | or | 33–40 | |||
| 86. 23. 34 | or | 85–36–34 | or 82°–17′ |
| No 80 | ° ′ | by Nepier | by Minto | ||||
 |
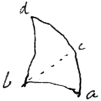given | a = | 59–47 | abc | = | 42–12–30 | 44– 2–19 |
| b = | 77–39–23 | cbd | = | 35–26–53 | 33–37– 3 | ||
| d = | 64–15. | ab | = | 50– 3 | 52–58– 0 | ||
| found | bd | = | 47–21 | 43–29–22 | |||
| ad | = | 56–15 | |||||
| No 81 | ° ′ ″ | found by Nepier | by Minto | |||
 |
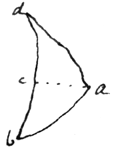given | a = | 112–40–45 | cad = | 65–43–45 | 64–31 |
| b = | 66– 7 | bac = | 46–57 | 48– 9–44 | ||
| d = | 59–50 | a = | 112–45–45 | 112–40–44 | ||
| ? | ab = | 65–34 | 66–38 | |||
| ad = | 74–20 | 73–54–59 | ||||
| bd = | 76–19–17 | 78–261 | ||||
| No 86. | ° ′ | found in ye opern | |||||
 |
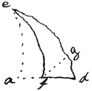given | de = | 79–56 | af = | 34–37–54″ | eg = | 62–34– 8 |
| ef = | 66–29 | ad = | 68–52–21 | dg = | 17–21–52 | ||
| df = | 34–14–27 | aef = | 38–17–58 | fg = | 29–59–24 | ||
| aed = | 71–19–40 | ||||||
| originally given | by Bezout | by Minto | |||||
| reqd | d = | 62–40 | 62–39– 6 | 62–39–47 | |||
| e = | 33– 2 | 33– 1–42 | 33– 1–46 | ||||
| f = | 72–32– 6 | 72–30–39 | 107–28–41 ? | ||||
| ae = | 61– 1– 0 |  |
32– 0–56 = dfg ? | ||||
| 75–27–45 = efg ? | |||||||
| give d. e. & f. as above to find de, ef, df |
|
by Minto, the results are of | ef = | 16– 1–34 by Bez. & Nepier |
| df = | 59–53–30 | |||
| eg = | 13–33–37 | |||
| dg. impossible | ||||
there must be something vicious in the data.
MS (CSmH: JF); written entirely in TJ’s hand on both sides of a single sheet.
In setting up these problems, TJ drew on examples published in John Potter, A System of Practical Mathematics (London, 1753; no. 3667), 277–8, 284–6, 289–90, 293. What TJ here calls the supplementary formula for solving spherical triangles when either all of the angles or all of the sides are known comes from David Steuart Erskine and Walter Minto, An Account of the Life, Writings, and Inventions of John Napier, of Merchiston (Perth, 1787), 97–103. For TJ’s explanation of Napier’s (nepier’s) Theorem, see TJ to Louis H. Girardin, 18 Mar. 1814, and Notes on Napier’s Theorem, printed above at 18 Mar. 1814. Étienne bezout discussed methods for solving spherical triangles given the values for the three sides in his Cours de Mathématiques, a l’usage Des Gardes du Pavillon et de la marine (Paris, 1764–69; no. 3680) pt. 2, 345–6, 349–50.
1. Recto ends here.
Index Entries
- An Account of the life, writings, and inventions of John Napier, of Merchiston (D. S. E. Buchan and W. Minto) search
- A System of Practical Mathematics (J. Potter) search
- Bézout, Étienne; Cours de Mathématiques, a l’usage Des Gardes du Pavillion et de la marine search
- books; on mathematics search
- Buchan, David Steuart Erskine, 11th Earl of; An account of the life, writings, and inventions of John Napier, of Merchiston search
- Cours de Mathématiques, a l’usage Des Gardes du Pavillion et de la marine (É. Bézout) search
- Jefferson, Thomas; Writings; Trigonometry Exercise search
- mathematics; books on search
- mathematics; Napier’s theorem search
- mathematics; trigonometry search
- Minto, Walter; An Account of the Life, Writings, and Inventions of John Napier, of Merchiston search
- Napier, John (of Merchiston); mathematical theorem of search
- Potter, John (mathematician); A System of Practical Mathematics search