John Winthrop to Benjamin Franklin: On Observing the Transit of Venus, 6 September 1769
From John Winthrop
AL (extract): The Royal Society
The observations of the transit of Venus in 1761 had not fulfilled the widespread hope of establishing the solar parallax, and thereby the mean distance between the earth and the sun. The hope grew, however, as scientists of many countries prepared to improve and expand their observations of the transit of June, 1769. Although John Winthrop was no longer well enough to play the active part that he had in 1761, he was deeply concerned not only with the collection of data on the second transit but also with the interpretation of them.5 In the letter below, sent via Franklin to the Royal Society, Winthrop wrestled analogically with the problem of the relationship between three moving bodies: for the passage of light he substituted that of a cannon ball; for the sun and its two satellites, Venus and the earth, he substituted a fort and two ships under sail.
Sept. 6. 1769
Extract of a Letter from John Winthrop, Esqr. Hollisian Professor of Mathematics and Natural Philosophy, at Cambridge N. England, to B. Franklin, LL.D., F.R.S. Dated Sept. 6. 17696.
I find that Mr. Bliss and Mr. Hornsby in their calculations in the Philos. Transact.7 suppose the phases of the Transit of Venus to be accelerated by the equation for the observation of light, which amounts to 55″ of time. According to my idea of aberration, I should think the Transit would be retarded by it. I can very easily suppose that I am in an error; and that I may more readily be led out of it, I beg leave to lay before you the several steps by which I have been led into it. And I think it will be best to take some similar instance, rather than to consider the thing in a general abstract manner.
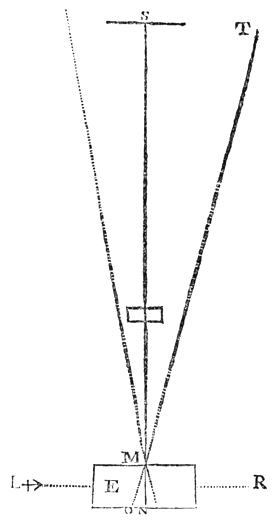 1. Let the parallelogram E represent a vessel sailing in the line to R, from left hand to right; and S, a fix’d Station, e.g. a castle, discharging balls on the right line SM, perpendicular to the route of the vessel. If the vessel had been at rest, a ball arriving at the middle of it, M, would have gone right across it, to N. But as it is supposed to be sailing, the ball will not go right over from M to N, but will cross the deck obliquely, in another right line as MO, and so will be left behind toward the stern as much as the vessel had gone forward while the ball was crossing it; and MN will be to NO as the velocity of the ball to the velocity of the vessel. Thus to the people on board, the ball would seem to move obliquely across the deck, as if it came from some point T in the line OM produced, instead of coming from S. And a tube capable of receiving the ball would allow the ball to pass thro it without striking its sides, if it were inclined forward in the direction OM; which it would not do in any other situation. The angle OMN or SMT answers to the aberration; and supposing S to be the Sun; and E, the Earth, this angle is 20″; and the general effect is, to make the Sun or any fixed star to appear farther that way towards which the Earth is moving.
1. Let the parallelogram E represent a vessel sailing in the line to R, from left hand to right; and S, a fix’d Station, e.g. a castle, discharging balls on the right line SM, perpendicular to the route of the vessel. If the vessel had been at rest, a ball arriving at the middle of it, M, would have gone right across it, to N. But as it is supposed to be sailing, the ball will not go right over from M to N, but will cross the deck obliquely, in another right line as MO, and so will be left behind toward the stern as much as the vessel had gone forward while the ball was crossing it; and MN will be to NO as the velocity of the ball to the velocity of the vessel. Thus to the people on board, the ball would seem to move obliquely across the deck, as if it came from some point T in the line OM produced, instead of coming from S. And a tube capable of receiving the ball would allow the ball to pass thro it without striking its sides, if it were inclined forward in the direction OM; which it would not do in any other situation. The angle OMN or SMT answers to the aberration; and supposing S to be the Sun; and E, the Earth, this angle is 20″; and the general effect is, to make the Sun or any fixed star to appear farther that way towards which the Earth is moving.
2. Let us suppose another vessel V, between S and E, sailing the same way as E in a parallel direction. If both the vessels sailed with the same velocity, a ball from V coming to M, would go right across to N, just as if both of them had been at rest; because the ball, which crossing the vessel E, would be carry’d just as far to the right hand as the points M and N are. And a tube to receive it must be held in the direction MN. So here would be no aberration of the vessel V.
because the ball, which crossing the vessel E, would be carry’d just as far to the right hand as the points M and N are. And a tube to receive it must be held in the direction MN. So here would be no aberration of the vessel V.
3. Suppose V to move the same way, but Slower. A ball from V would now be really carry’d forward, that is, to the right hand, tho not so far as in the 2d. supposition; and therefore would be left behind in respect of the vessel E; and so, would come to the side of the vessel somewhere between O and N, but the greater its velocity towards the right, the nearer to N. So that if the velocity of V were to be continually increasing from nothing till it became equal to that of E, a tube to receive the ball must be held first in the direction OM looking forward, and afterwards, more and more inclined till it came into the perpendicular direction. From hence it is natural to conclude,
4. That if V move the same way, but swifter, a tube to receive the ball must be reclined backward. For the ball would now be carried to the right hand farther than in the 2d supposition; and therefore would come to the other side of the vessel at some point P on the right hand of N, as if it proceded from some point Q on the left hand of S.
This last seems to be the case of the Transit, by supposing S to be the Sun, E the Earth; and V the planet Venus passing between them, from left to right, and with a greater velocity than the Earth; (greater, nearly as 24:20.). And it should seem that the aberration must make Venus appear farther to the left hand, or to the East from the Sun, and consequently retard the Transit, and make it happen later than it would otherwise do.
Thus, Sir, I have explain’d very particularly my apprehension of the matter, and I make no doubt you will immediately discover where the error lies; and shall take it as a great favor if you will please to point it out to me.
5. For the part he did play in attempting, unsuccessfully, to persuade Massachusetts to send an expedition see above, XV, 167. He also did what he could to arouse public interest: talks that he gave at Harvard subsequently appeared in print as Two Lectures on the Parallax and Distance of the Sun as Deducible from the Transit of Venus … Published by the General Desire of the Students (Boston, 1769), with an appendix in which the layman was shown how to make his own observation of the transit.
6. In BF’s hand. The paper, read on April 5, 1770, was printed in Phil. Trans., LX (1770), 358–62.
7. Nathaniel Bliss (1700–64) was Savilian Professor of Geometry at Oxford and became Astronomer Royal in 1762; the Rev. Thomas Hornsby (1733–1810) became Savilian Professor of Astronomy in 1763. DNB. Winthrop is probably referring to Bliss’s paper on the transit of 1761 in Phil. Trans., LIT, pt. I (1761), 232–50, and to Hornsby’s on that of 1769 in ibid., LV (1766), 326–44.

![University of Virginia Press [link will open in a new window] University of Virginia Press](/lib/media/rotunda-white-on-blue.png)
