Calculation of the longitude of the Capitol, in the city of Washington, from Greenwich observatory, in England, from the beginning of the Solar Eclipse of August 27th 1821, Examined and revised.

---

---

	°	′	″	dec 1
Latitude of the Capitol, by a mean of 21 observations	38.	52.	45.	—
Do     do   reduced, (320 to 319)	38.	42.	14.	51.
Obliquity of the Ecliptic,	23.	27.	56.	50.
Moon’s longitude, (Naut. Alm.)	152.	31.	7.	39.
〃 true latitude, North descending	0.	11.	41.	70.

---

---

		h.	m	Sec.	d			°	′	″	dec
Beginning of the Eclipse, Augt 26th		19.	22.	4.	50.	=		290.	31.	7.	50.
Estimated longitude, West	+	5.	7.	42	—
Corresponding time at Greenwch 27th		0.	29.	46.	50	☉’s R.A.		155.	44.	54.	35
Right ascension of the meridian from beginning of ♈,								86.	16.	1.	85.
Do     do        from beg: of ♑,								176.	16.	1.	85.

 

---

---

Method I.

 

With the Moon’s longitude, = 152.° 31.′ 7.″ 39 dec., true lat. N. 0.° 11.′ 41.″ 70. dec and the obliquity of the Ecliptic, 23.° 27.′ 56.″ 50 dec, compute the Moon’s right ascension, = 154.° 33.′ 49.″ 98 dec, and declination, North, 10.° 46.′ 13.″ 14. dec

Find the angle between the parallels to the Ecliptic and Equator,[✴] = 21.° 4.′ 32.″ 80 dec, which added to 90°, gives 111.° 4.′ 32.″ 80 dec, the angle between the meridian passing thro’ the Moon’s center, and a parallel to the Ecliptic.

From the Moon’s Right ascension, subtract the right ascension of the meridian from ♈, the remainder, = 68.° 17.′ 48.″ 13 dec, is the Moon’s horary angle, or distance from the meridian, East.

With this angle, − the Moon’s declination, and the latitude of the place reduced, compute the Moon’s true altitude, = 23.° 36.′ 1.″ 18 dec., and angle of position, = 52.° 18.′ 7.″ 34. dec

		°	′	″	dec
Angle between merid. passing thro’ ☽’s center, and par: to Eclip:		111.	4.	32.	80.
Angle of position, (☽ East of meridian)	+	52.	18.	7.	34.
Angle between the vertical circle, and a par: to ecliptic		163.	22.	40.	14.

With the Moon’s hor: parallax à ☉, = 0.° 55.′ 4.″ 75 dec, and true altitude, = 23.° 36.′ 1.″ 18 dec, compute the parallax in altitude, = 0.° 50.′ 47.″ 54 dec, which subtracted from the true altitude, gives 22.° 45.′ 13.″ 64 dec, the Moon’s apparent altitude, exclusive of refraction.

 

For the parallax in latitude.

	°	′	″	dec
Moon’s hor: parallax à ☉,	0.	55.	4.	75		Sine		8.2046948.
〃  apparent alt:	22.	45.	13.	64		Cosine		9.9648135.
Angle between Vert. circle and p. to E.	163.	22.	40.	14		Sine		9.4564562.
Parallax in lat. found nearly	0.	14.	31.	75		Sine		7.6259645
Moon’s true lat. N.	0.	11.	41.	70
〃 apparent lat. S. found nearly	0.	2.	50.	05.

 

For the parallax in longitude.

	°	′	″	dec
Moon’s hor. parallax à ☉,	0.	55.	4.	75		Sine		8.2046948.
〃  apparent altitude,	22.	45.	13.	64		Cosine,		9.9648135.
Angle bet: vert. circle, and pl to ecl:	163.	22.	40.	14		Cosine,		9.9814616.
Moon’s apparent lat. found nearly	0.	2.	50.	05		ar: comp. cosine,		0.0000001.
Parallax in longitude,	0.	48.	40.	18		Sine		8.1509700.

 

Correction of the Moon’s apparent latitude.

 

Constant log. (one fourth of the radius in sec. 51566.″)							4.7124.
2 × Sine parallax in longitude,							16.3019.
Sine of twice Moon’s true lat. = 0.° 23.′ 23.″ 40 dec							7.8327.
				″	dec
[☞]	Correction, +			0.	07		8.8470.2
Moon’s app. lat. S. nearly,			2.	50.	05.
Moon’s app: lat. S. correct,		0.	2.	50.	12.3

 

---

---

Method II.

 

With the altitude of the nonagesimal, = 74.° 43.′ 21.″ 03. dec the Moon’s distance from the north pole of the ecliptic, = 89.° 48.′ 18.″ 30. dec and true distance from the nonag. = 65.° 32.′ 15.″ 87 dec, find the parallactic angle, = 73.° 22.′ 40.″ 14 dec, and the Moon’s true altitude, 23.° 36.′ 1.″ 18. dec

Find the Moon’s parallax in altitude, and apparent altitude, which will be the same as before. The process for the parallaxes in latitude and longitude, is to be varied by using the log. cosine of the parallactic angle, in the former, and its log. sine in the latter: the cosine of 73.° 22.′ 40.″ 14. dec being equal to the sine of 163.° 22.′ 40.″ 14 dec, and the Sine of the first, to the co-sine of the other arch. As the same results will be produced, it is unnecessary to repeat the operation.

 

---

---

Method III.

 

It is customary for some astronomical calculators to find the parallax in longitude by approximation, and to extend it to the second only; this is not sufficiently correct. To have it so, we should carry it to the fourth approximation, and then the parallax in longitude will be accurately ascertained.

			°	′	″	dec
Moon’s hor: par: à ☉,			0.	55.	4.	75		Sine,		8.2046948.
Altitude of the nonag:			74.	43.	21.	03		Sine		9.9843747.
Moon’s true latitude, N.			0.	11.	41.	70.		ar: comp. cos:		0.0000025
								Sine		8.1890720.	(a)
Moon’s true distance à nonag:			65.	32.	15.	87		Sine		9.9591532.	(b)
1st approximation,	a+b,		0.	48.	21.	78		Sine		8.1482252.	(c)
	b+c		66.	20.	37.	65		Sine,		9.9618811.	(d)
2d approximation,	a+d		0.	48.	40.	06		Sine,		8.1509531.	(e)
	b+e,		66.	20.	55.	93		Sine		9.9618979.	(f)
3d approximation,	a+f		0.	48.	40.	18		Sine		8.1509699.	(g)4
	b+g		66.	20.	56.	05.		Sine		9.9618980.	(h)
Parallax in longitude,	a+h,		0.	48.	40.	18		Sine,		8.1509700.	(i)

 

The above process by approximation is tedious; the following rule is attended with less labor, and will always5 give a correct result.

(a) found as above			8.1890720.
Moon’s true distance à nonag:		65.° 32.′ 15.″ 87.	cosine, 9.6170987.
Corresponding natural number,		0063998.	(A) log., 7.8061707.
Natural number, A,			0063998.
			ar. comp. 9936002.
Corresponding log.		9.9972117
arith: comp:		0.0027883.
(a)		8.1890720.
Moon’s true dist. à nonag.		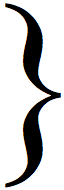9.9591532.
Sine
tangent,		8.1510135.	par: in long: 0.° 48.′ 40.″ 18. dec

 

For the Moon’s apparent latitude.

			°	′	″	dec
Moon’s hor: par: à ☉,			0.	55.	4.	75		Sine,	8.2046948.
Altitude of the nonages:			74.	43.	21.	03		cosine	9.4207709
Corresponding nat. number,		0042215						(B)	7.6254657.
Nat: Sine Moon’s true lat,		0034019.
(C) South,		0008196						log.	6.9136019

		°	′	″
Moon’s true distance à nonag:		65.	32.	15.	87.	ar. comp. S.	0.0408468.
〃 apparent dist: do		66.	20.	56.	05	Sine	9.9618980.
〃 true latitude,		0.	11.	41.	70.	ar. co: cos.	0.0000025.
〃 apparent lat. S.	+	0.	2.	50.	12.	tang.	6.9163492.
Parallax in lat. correct.		0.	14.	31.	82.

 

The accuracy of the last result will be tested by Doctr Maskelyne’s rule, assuming the Moon’s apparent latitude to be 0.° 2.′ 50.″ 12. dec

		°	′	″
Moon’s hor: parallax à ☉,		0.	55.	4.	75		Sine,	8.2046948.
Altitude of the nonag:		74.	43.	21.	03		cosine	9.4207709.
Moon’s app: lat. S. (assumed)		0.	2.	50.	12		cosine,	9.9999999.
1st part parallax in latitude		0.	14.	30.	75		Sine	7.6254656.
Moon’s hor: parallax à ☉,		0.	55.	4.	75		Sine	8.2046948
Altitude of the nonagesimal,		74.	43.	21.	03		Sine	9.9843747.
Moon’s apparent lat. (assumed)		0.	2.	50.	12		Sine	6.9160545
〃 true dist. à nonag: + par: in long/2		65.	56.	38.	66		cosine	9.6102642.
2d part par: in latitude		0.	0.	1.	07		Sine,	4.7153882.
1st part	+	0.	14.	30.	75.
parallax in lat.		0.	14.	31.	82.

 

To find the Moon’s augmented Semidiameter.

			°	′	″	dec
Moon’s true zenith distance,			66.	23.	58.	82.	ar: comp: sine,	0.0379337.
	〃 apparent  do		67.	14.	46.	36	Sine	9.9648135.
	〃 horizontal Semr		0.	15.	4	—	Sine	7.6417419.
	〃 augmented Semidr		0.	15.	9.	74.	Sine	7.6444891.
	Inflection of light,	−	0.	0.	2.	98.
	Semidr corrected,		0.	15.	6.	76.

		″	dec
Sun’s Semidiameter,		952.	15.
〃 irradiation of light,	−	1.	62
〃 Semidiamr corrected,		950.	53.
Moon’s  do    do		906.	76
Sum,		1857.	29
Moon’s apparent lat.		170.	12
Sum,		2027.	41		log.		3.3069415.
		1687.	17		log.		3.2271589.
						2)	6.5341004.
							3.2670502.
Moon’s apparent lat: arith. comp: cosine							0.0000002.

diff: ☉ and ☽’s apparent long.

}

30.′ 49.″ 48 dec

=

1849.″ 48 dec

log.

3.2670504.

			°	′	″	dec
	Sun’s longitude at beginning of the Eclipse,		153.	50.	37.	00.
	Parallax in longitude,	−	0.	48.	40.	18.
	Diff: of ☉ and ☽’s apparent longitude,	−	0.	30.	49.	48.
	True longitude ☽’s center, by calculation,		152.	31.	7.	34.
Apparent time at Greenwich, when the Moon had that long:			0.	29.	46.	42.
〃	of the beginning of Eclipse at Washington		19.	22.	4.	50.
Longitude, in time,			5.	7.	41.	92.
	Equal to 76.° 55.′ 28.″ 80. dec

This result differs 60⁄100 of a second from that contained in my report, page 64, which, in the latitude of the capitol in Washington, amounts to 15 yards, 2 feet, 5 inches, if taken singly; when combined with the other results, page 79, the variance amounts to 10⁄100 of a second, = 2 yards, 1 f: 11 inches, nearly.

 

---

---

To find the parallactic angle, and Moon’s true altitude.

		°	′	″	dec
Altitude of the nonagesimal,		74.	43.	21.	03	tangent,	10.5636038.
Moon’s true dist. à nonag.		65.	32.	15.	87.	cosine,	9.6170987.
arch I.		56.	35.	24.	48	tang:	10.1807025
Moon’s dist. from N. pole Eclip:		89.	48.	18.	30.
arch II,		33.	12.	53.	82	cosecant,	10.2613926.
arch I,		56.	35.	24.	48	Sine	9.9215580.
Moon’s true dist. à nonag.		65.	32.	15.	87	tangent	10.3420545.
Parallactic angle,		73.	22.	40.	10	tangent,	10.5250051.
Arch II,		33.	12.	53.	82	cotangent,	10.1839216.
Parallactic angle,		73.	22.	40.	10	cosine,	9.4564565
Moon’s true altitude,		23.	36.	1.	18.	tangent,	9.6403781.

Rule II.

	°	′	″	dec
Moon’s true dist. à nonag:	65.	32.	15.	87		log. versed Sine,		9.7678282.
Moon’s dist: from N. pole Ecl:	89.	48.	18.	30		Sine		9.9999975
Altitude of the nonagesimal,	74.	43.	21.	03		Sine		9.9843747.
diff: = Arch A.	15.	4.	57.	27.		Secant		10.0152244.
arch B.	65.	30.	12.	60.		Versed Sine		9.7674248.
Arch A,						Secant,		10.0152244.
B,						Secant,		10.3823312.
	°	′	″	dec
Moon’s true altitude,	23.	36.	1.	17.		Cosecant,		10.3975556.
	90	—	—	—
Moon’s true zenith dist:	66.	23.	58.	83,		ar: comp. sine,		0.0379337.
〃 true dist. à nonag.	65.	32.	15.	87		Sine,		9.9591532.
Altitude of the nonag:	74.	43.	21.	03		Sine		9.9843747.
Parallactic angle,	73.	22.	40.	17		Sine,		9.9814616.
By rule 1,	73.	22.	40.	10
Mean result,	73.	22.	40.	13.

☞  The small variance in these elements would not affect the Moon’s parallaxes in longitude and latitude, 1⁄500 part of a second.

When the original calculation of the longitude, by this eclipse was made, the author was too much occupied with observations of the Moon’s transits over the meridian at the Capitol, in Washington, and the calculations founded thereon, to examine and revise it at that time. It occurred to him lately, that a small error had been committed in the Moon’s parallaxes in longitude and latitude, and that determined him to employ more than one method to ascertain those elements with due precision. The first method is wholly independent of the altitude and longitude of the nonagesimal: the Second, by finding the parallactic angle and Moon’s true altitude, confirms the accuracy, or points out the errors that may have been made in the first, and both are a never failing check on the results found by the third method, which is the one generally used by astronomical calculators.

The angle of position referred to in this communication, is the angle opposite to the complement to 90.° of the latitude of the place, reduced; and to find it, in this case, we have the contained angle, = the Moon’s horary angle, 68.° 17.′ 48.″ 13. dec and two sides of an oblique angled spherical triangle, viz: the Moon’s North polar distance, = 79.° 13.′ 46.″ 86 dec, and complement of the latitude of the Capitol, in Washington, reduced, = 51.° 17.′ 45.″ 49 dec, to find the other side, = the Moon’s true zenith distance, 66.° 23.′ 58.″ 82 dec, and the angle of position opposite to the second side, = 52.° 18.′ 7.″ 34 dec. The third angle, = Moon’s true azimuth from the North, 95.° 5.′ 30.″ 74. or 84.° 54.′ 29.″ 26. dec from the South, not being wanted in the calculation of the Moon’s parallaxes. This last angle is opposite to the Moon’s north polar distance.